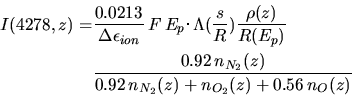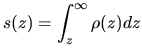
Next: Artificial airglow Up: Aurora and artificial airglow Previous: (F)our Auroral lines Contents
Modelling of the emissions
To model the spatial distribution and temporal variation of the
emissions it is necessary to model accurately the contribution from
the different sources. Since there are both direct electron
excitation of the excited states and chemical sources with temperature dependent reaction rates, a model of the emission
must account for the chemistry and thermodynamics of the thermosphere
as well as the altitude and energy variations of the energetic
electron flux. To do this it is necessary to solve the continuity
equations of the minor neutral species
![]() and the ions
and the ions
![]() in order to obtain the time- and altitude-dependent variations in
the ionosphere-thermosphere system. Further it is necessary to solve
the neutral, ion, and electron energy equations in order to
obtain the variations in the temperatures. In order to take the
electron excitation into account it is also necessary to model the
electron transport and production of secondary electrons. This gives a
coupled set of non-linear partial differential equations which must be
solved simultaneously. Precipitating electrons ionize the atmosphere
along their trajectories, creating secondary electrons with lower
energies. This process increases the electron and ion concentrations
and, moreover, leads to heating of the ions and ambient electrons
through energy dissipation. From the ion-neutral collisions the
neutral atmosphere is heated, the change in the temperatures
influence the chemistry that affects the recombination rates of
electrons and ions. The electron transport alone is a complicated
process -- expressed by a
continuity equation taking into account discrete and continuous energy
loss processes, scattering of energetic electrons and production of
secondary electrons by ionization. A more detailed description of this
interesting and challenging science is outside the scope of this
thesis.
in order to obtain the time- and altitude-dependent variations in
the ionosphere-thermosphere system. Further it is necessary to solve
the neutral, ion, and electron energy equations in order to
obtain the variations in the temperatures. In order to take the
electron excitation into account it is also necessary to model the
electron transport and production of secondary electrons. This gives a
coupled set of non-linear partial differential equations which must be
solved simultaneously. Precipitating electrons ionize the atmosphere
along their trajectories, creating secondary electrons with lower
energies. This process increases the electron and ion concentrations
and, moreover, leads to heating of the ions and ambient electrons
through energy dissipation. From the ion-neutral collisions the
neutral atmosphere is heated, the change in the temperatures
influence the chemistry that affects the recombination rates of
electrons and ions. The electron transport alone is a complicated
process -- expressed by a
continuity equation taking into account discrete and continuous energy
loss processes, scattering of energetic electrons and production of
secondary electrons by ionization. A more detailed description of this
interesting and challenging science is outside the scope of this
thesis.
Several ionosphere-thermosphere-electron-transport models exist in the scientific community. The following list of references is intended to be neither complete nor fair, but should rather be seen as a set of starting points for reading:
- An exhaustive overview of the factors that influence the auroral ionosphere-thermosphere is given by Roble and Rees (1977).
- A detailed electron transport code is presented by Lummerzheim and Lilensten (1994).
- A coupled electron-hydrogen-proton transport model including the effects of proton precipitation is reported and used by Basu et al. (1993) and Strickland et al. (1993).
- A pseudo three-dimensional model for the ionosphere is the USU (Utah State Univ.) time-dependent ionospheric model (TDIM) in which the one-dimensional ion and electron continuity equations are solved as functions of height for convecting flux tubes ( Schunk, 1988).
- Solomon et al. (1988) and Hill et al. (2000) present work based on a model of one-dimensional electron transport coupled to the chemistry.
- The electron transport problem has been solved with Monte Carlo methods by e.g. Ivanov and Sergienko (1992).
The ionospheric modeling has been used to derive methods to make
estimates of the characteristics of the precipitating particles from
optical observations. Rees and Luckey (1974) derived relations between the
intensities of the emissions in 5577 Å, 4278 Å and 6300 Å
and the total fluxes and characteristic energies of the precipitating
electrons. Strickland et al. (1989) addressed the problem of
variability in oxygen density and suggested an improved method which
derived electron characteristics and oxygen scaling factors from
ratios of the emissions at 4278 Å, 6300 Å and 7444
Å. This method was then extended to use the 8446 Å emission
instead of the 7444 Å. Use of the 6300 Å emission
requires stable conditions to give reliable results,
because of the long effective lifetime of ![]() . Rapid variations
in precipitation give rapid changes in the emissions in the other
wavelengths but the 6300 Å emission is smoothed.
. Rapid variations
in precipitation give rapid changes in the emissions in the other
wavelengths but the 6300 Å emission is smoothed.
Further, the altitude distribution of the emission depends on the
characteristic energy of the precipitating electrons. This has been
used to derive characteristics of the precipitating electrons
e.g. Aso et al. (1998a). Since the ![]() state has several
sources (c.f. section 2.1), the retrieval of
the spectra of the primary electrons is impaired by uncertainties that
are difficult to overcome. Retrieval of the primary electron spectra
from an emission with only one signifficant source such as 4278 Å
is a simpler problem. If we use the experimentally derived electron
transport relations presented in Rees (1989) with energy deposition,
scattering depth, effective range, and an average energy loss per
ionization, the retrieval of the primary spectra becomes a linear
inverse problem if we assume that 2.13 % of the
state has several
sources (c.f. section 2.1), the retrieval of
the spectra of the primary electrons is impaired by uncertainties that
are difficult to overcome. Retrieval of the primary electron spectra
from an emission with only one signifficant source such as 4278 Å
is a simpler problem. If we use the experimentally derived electron
transport relations presented in Rees (1989) with energy deposition,
scattering depth, effective range, and an average energy loss per
ionization, the retrieval of the primary spectra becomes a linear
inverse problem if we assume that 2.13 % of the ![]() ionization
leads to emission in 4278 Å. The emission caused by a mono-energetic
electron beam with energy
ionization
leads to emission in 4278 Å. The emission caused by a mono-energetic
electron beam with energy ![]() in
in ![]() in 4278 Å at
altitude
in 4278 Å at
altitude ![]() is given by:
is given by:
Here
 |
where
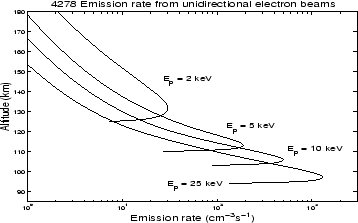 |
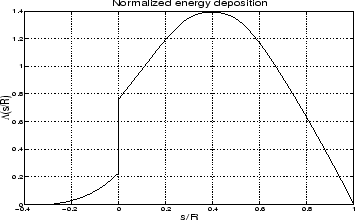
With increasing incident electron energy the altitude variation of the emission changes to peak at lower altitudes with decreasing widths, as shown in Figure 2.3. In paper II this method was used to determine the incident electron spectra for a 20 minute event with a stable auroral arc.
Next: Artificial airglow Up: Aurora and artificial airglow Previous: (F)our Auroral lines Contents
copyright Björn Gustavsson 2000-10-24